Simulation Bridge Dynamic Self-Weight Stress Analysis

Here we present a simulation of a bridge considering a dynamic self-weight stress analysis. The bridge is modeled as a linear elastic material, which means Hooke's law is applicable. The computation starts with an initial geometry created using the CAD software. Due to its self-weight the bridge starts to deform. Due to these large deformations, motion and acceleration can no longer be neglected, as would be the case in the infinitesimal strain theory. Therefore, Newton's laws must be applied - the force acting on the body results in proportional acceleration, making a time-dependent analysis necessary.

Even though we used a linear elastic material in this case study, NOGRID software is also capable of computing viscoelastic materials as well. Unlike purely elastic bodies, viscoelastic materials exhibit both elastic and viscous behavior. The viscosity of a viscoelastic material gives the body a time-dependent strain rate. Purely elastic materials do not dissipate energy when a load is applied and then removed. In contrast, viscoelastic materials do dissipate energy under the same conditions.

Viscoelastic behavior has elastic and viscous components modeled as linear combinations of springs and dashpots, respectively. Each model differs in the arrangement of these elements. The elastic components can be modeled as springs of elastic constant E, given the formula:

σ = E ε

where σ is the stress, E is the elastic modulus of the material, and ε is the strain that occurs under the given stress, similar to Hooke's law. The viscous components can be modeled as dashpots such that the stress–strain rate relationship can be given as,

σ = η dε/dt

where σ is the stress, η is the viscosity of the material, and dε/dt is the time derivative of strain.

CAD model bridge created in NOGRID's COMPASS

Figure 1: CAD groups created in NOGRID's COMPASS

Bridge stress analysis setup within NOGRID points CFD software GUI

Figure 2: Setup the bridge stress analysis case

Bridge stress analysis NOGRID points CFD software GUI results

Figure 3: Result view of the bridge stress case within NOGRID points' GUI

Animation displacement bridge by self-weight load, displayed in NOGRID points CFD software

Figure 4: Animation displacement by self-weight load

The implemented Maxwell model can be represented by a purely viscous damper and a purely elastic spring connected in series. According to this model, if the material is subjected to a constant strain, the stress gradually relaxes over time. When a material is subjected to a constant stress, the resulting strain has two components: First, an elastic component occurs instantaneously - corresponding to the spring - and relaxes immediately upon release of the stress. Second, a viscous component develops gradually and continues to increase as long as the stress is applied.

The implemented Kelvin–Voigt model consists of a Newtonian damper and a Hookean elastic spring connected in parallel. This model represents a solid undergoing reversible, viscoelastic strain. When a constant stress is applied, the material deforms at a decreasing rate, asymptotically approaching a steady-state strain. Once the stress is removed, the material gradually returns to its undeformed state. If the viscous stress is absent (η=0), the material behaves as a purely elastic body, and only Hooke's law applies.

In addition, the Generalized Maxwell model - also known as the Wiechert model - is implemented as well. It represents the most general form of the linear viscoelastic model. This model takes into account that the relaxation does not occur at a characteristic time, but over a distribution of times.

The Generalized Maxwell model consists of one or more Maxwell elements (each comprising a viscous damper and an elastic spring connected in series), optionally combined with a purely viscous element and/or a purely elastic element, all connected in parallel. A special deduction of the Generalized Maxwell model implemented in NOGRID software is the Tool-Narayanaswamy-Moynihan model.

NOGRID points can be used for designing and problem solving for all kinds of stress related tasks.

Capabilities of NOGRID points CFD software

NOGRID points combines the capability to handle stress computations for large deformations and various viscoelastic materials, allowing the simulation of any conceivable geometry and operating mode, such as

fully 3D computation solving viscoelastic material behavior
easy and intuitive setup
freely definable material properties by user equations or user-defined curves
Structure-structure interaction with viscoelastic materials
Fluid-structure interaction using all implemented viscoelastic models
computation of moving parts or moving boundaries in combination with viscoelastic materials

Whitepaper Nogrid points Technology

Nogrid's strengths

Particular strengths of NOGRID's fluid flow CFD software are the rapid preprocessing (no fluid grid needs to be generated, only the boundary mesh, inner finite points are generated automatically depending on User setting initially and during computation), and the outstandingly short computation time even for complicated cavities.

As you can see in the image below, the boundary of the geometry still requires a mesh to allow the interior finite points to detect the boundary. The boundary must therefore be meshed and the finite points inside are automatically generated during the simulation controlled by User specifications.

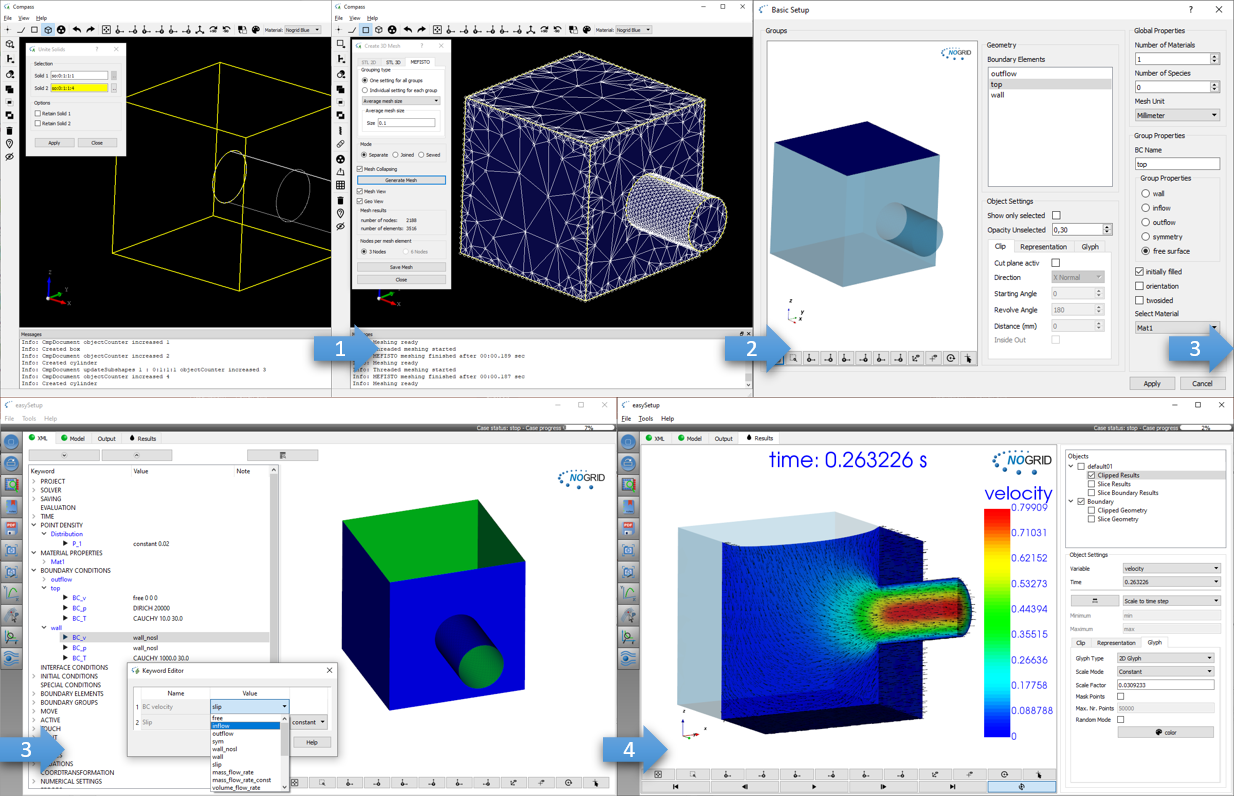
Easy and fast modelling: Build geometry, mesh boundary, setup the case and start computation

What is CFD from NOGRID?

CFD solves the fundamental equations that define the fluid flow process. With CFD software from NOGRID every engineer makes better decisions by predicting, analyzing and controlling fluid flow, heat and mass transfer or chemical reaction. By using NOGRID software for flow modeling you receive information on essential flow characteristics as for example flow distribution. Using it additional to testing and experimentation NOGRID software helps to improve the evaluation of your design – resulting in better construction and operation parameters, increasing planning security and money savings due to faster time to the marketplace for your product or process.

Choose NOGRID

With NOGRID, you choose professional CFD software and services – our aim is helping you to be successful. When you decide to work with NOGRID you choose close cooperation with a dynamic, flat hierarchies-organization. Short information channels result in quick and accurate professional support and service. Our team consists of highly qualified employees, who are experts in fields such as numerical simulation or computational fluid dynamics. Based on our know-how, we are pleased to offer the following services, responding to your individual requirements:

TRAINING

In our two-days training courses you will learn, how to use NOGRID CFD software efficiently. Our technical support team will teach you how to handle and solve different cases.

For more details please refer to Training Courses →

Technical Support

We offer full professional support from the minute you start using our software, by telephone or by email. Contact us, when ever needed.

For more details please refer to Software Support →

Service

Lack of time or resources and other constraints often make outsourcing an attractive solution. We help you with your flow modeling needs. Based on our engineering expertise in this field we offer individual numerical simulation services matching the unique needs of your organization.

For more details please refer to Simulation Services →